Cantor-like sets

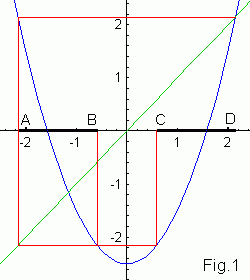
Cantor strange repeller

You see in Fig.1 that for c < -2 interval (BC) is mapped outside invariant interval I_c and all points go eventually to infinity. Two intervals [AB] and [BC] are mapped onto I_c. So similar to the tent map Cantor strange repeller with zero measure appears in quadratic maps (you see below such repeller for c = -3 on complex dynamical plane).

Further you see this repeller on (x, c) plane (for different c).

"Period three implies chaos"

In 1975 T.Y.Li and J.A.Yorke published the famous "Period three implies chaos" paper. It turns out that nonlinear 1D map with period-3 orbit has continuum of chaotic orbits. Let a,b,c make period-3 cycle
f(a) = b, f(b) = c, f(c) = a.
It follows from the Fig.2 that inverse function f(x)^-1 is multivalued in (b,c). When iterated f(x)^-1 value gets in this interval we can chouse any branch at random and make chaotic orbits.

Sharkovskii's theorem

Moreover if a map has period-3 orbit then it has orbits with every period. It is particular case of Sharkovskii's theorem that a map with period-n orbit has orbits with all periods n' preceding n in the list
1 < 2 < 2² < 2³ < ... < 2²7 < 2²5 < 2²3 < ... < 2·7 < 2·5 < 2·3 < ... < 7 < 5 < 3
where ... 7 5 3 are odd numbers.

Stable and unstable period-p cycles for quadratic map appear after tangent bifurcation of the f^op(x) map. With decreasing c stable cycle loses its stability and two period-2p cycles appear. Unstable cycles never die. Therefore after period doubling cascade completion quadratic maps have infinite set of unstable periodic orbits.

Cantor strange repeller in regular dynamics window

Therefore we meet complicated Cantor-like structures for c = -1.7542 corresponding to period-3 window of regular dynamics. For almost all x in interval I_c points are attracted to period-3 orbits (these points lie in circles). All the rest points (after cutting these circles) make Cantor strange repeller with zero measure. It includes unstable periodic orbits and chaotic continuum.

This regular map (with attracting period-3 orbit) is unpredictable in some way. The map f_c^o3 has 3 attracting fixed points. In the picture J(0)-midgets attracted to the same fixed point are colored in the same color. There is a funny "traffic lights" rule: in any interval, between two biggest midgets with different colors one more biggest circle has the third color (red, blue and green in the picture). Therefore all colors are dense in the I_c. You see, that basins of attraction of different fixed points are tightly interwoven again.

So the regular map has sensitive dependence on initial conditions as since you can find two arbitrary close points which diverge under iterations (asymptotically they go to the same cycle but with different "phases").

The basic dichotomy for real quadratic maps

For almost every c in [-2, 1/4], the quadratic map f_c: x → x² + c is either regular or stochastic [1]
For quadratic maps it is proven that the set of c values for which attractor is chaotic has positive Lebesque measure and attracting periodic orbits are dense in the set. I.e. between any two chaotic parameter values there is always a periodic interval.

"Fat" Cantor sets

We will get a general Cantor set if in the "1/3 cutting" process we cut the central 1/3 piece, then i.g. 1/9, then 1/27, etc. Resulting set is topologically equivalent to the standard Cantor set, but as since holes decrease in size very fast therefore the "fat" Cantor set has positive Lebesque measure and fractal dimension 1 .
In the real interval -2 < c <1/4 , regions with chaotic dynamics have nonzero Lebesgue measure and make a "fat" Cantor set. You can see below that regular dynamics regions (black M-midgets) are dense on complex plane along the real axis.

Amazingly regular dynamics windows (marked by m3,5,6...) for quartic maps (the lower picture) are ordered in the same way.
You can test density and periods of M-midgets by animated Cantor trip.

[1] Mikhail Lyubich The Quadratic Family as a Qualitatively Solvable Model of Chaos Notices of the AMS, 47, 1042-1052 (2000)

Contents Previous: Chaotic quadratic maps Next: "Transient" chaos
updated 8 Nov 06